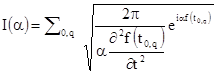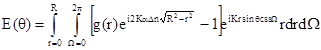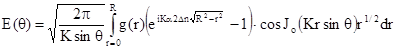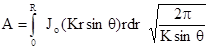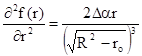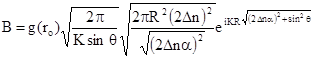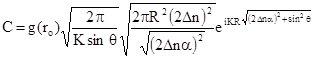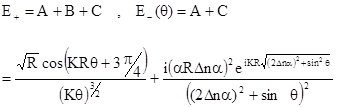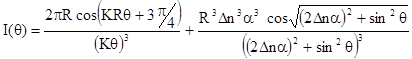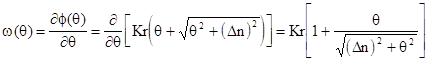Particle sizing methods – a stationary phase based comparison

The electromagnetic field at a distant point created by an object illuminated by a coherent beam, may be described as composed of many field elements, each having the form of a spherical wave:

[1]   

where v is the frequency of the impinging monochromatic light and l is its wavelength. Each of these field elements propagates through the object with wavelength corresponding to its immediate surrounding. This old idea, introduced in its primary form by Huygens, and thereafter validated by Kirchoff and others has been successfully used since.

Yet the problem of summing up small field elements (wavelets) may be found to be a difficult analytical problem. As mentioned, upon changing refraction index of the medium through which the wavelet travels, its wavelength should be correspondingly changed. Therefore, if a non-homogeneous object is dealt with, or in case its geometry is complicated, even integrals such as in may not be easy to solve. In case small scattering angles are of main interest, meaning that the distant detector is assumed to be located in angles q not larger than those defined by sin(q) q, then even a simpler expression exists by which angular intensity distribution of the field may be calculated, namely:

[2]

where D(r), Dn(r) correspondingly are the radial thickness and refraction index of the object at a distance r from the center. The angles q and f stand for scattering directions corresponding to the x and y axes (Fig. 1). Integration is performed over the boundary defined by the object cross section. The exact angular range in which the integral in [2] is adequate, is given by:

[3]

where D is the typical thickness of the object.

A few approaches for obtaining the scattering integral solution in [2] exist: (1) by numerical calculation, or (2) by use of expansions of the exponent so that a serial solution is obtained .

The so-called stationary phase approximation method seems to bypass this problem. Given an integral which involves a complex exponent variable of the form:

[4]

According to the stationary phase theorem, in case one may approximate I(a) by the expression:

[5]

where t₀ is the extreme point of obtained by solving the equation . The physical interpretation of [5] is, that the phasor sum needed in order to obtain a closed form of the scattered field, may be replaced by a more convenient one, which requires only finding a dominant wavelet. Being a phasor, this field wavelet has its own amplitude and phase at a given point. The reason one assumes such a wavelet exists, is based on the fact that when long optical paths are involved the typical phase acquired is large (the demand that), therefore any small difference between two neighboring paths will cause substantial phase difference. The results show that when summing over all the different paths connecting the wave front and a specific point of interest, most wavelets will have a negligible effect because their phase shifts are widely spread. Each phasor in the sum will be cancelled by an adjacent one having an opposite phase. However, if a group of paths exist with an almost identical optical length, their phasor sum will not be negligible and will therefore determine the value of the field at this point. Naturally, the quantity of these special paths will determine the field amplitude and their average phase will be the field local phase. The algorithm defined in [5] may be used in obtaining approximated solutions to scattering problems. A simple example is that of scattering pattern created by a dilute spheroidal particle of radius R. The phase shift j(r) and thickness g(r) of a sphere at a given distance from its center are given by:

[6 ]

The scattering integral of a sphere is written according to Eq. [2] in the following form:

[7]

where a polar coordinate system was used. Integration with respect to f yields:

[8]

Defining the sum:

[9] E(q) = A +B + C

where:

[10]

[11]

[12]

wehre J­_o(x) is approximated by its asymptotic form, and in case the physical terms are such that for each r < R - e :

[13]

then E_A(q) may be approximated according to stationary phase theorem by:

[14]

In order to get the approximated expressions for B and C, one should solve:

[15]

with the solution:

[16]

corresponding to B and C components in Eq. 14. Substitution of these results in Eq. 5 yields:

[17]

These solutions represent the field scattered to positive and negative angles:

[18]

so that the intensity of the light scattered to the positive zone is:

[19] I(q) = E(q)*E(q)=

Using this method, a separation is made between diffraction and refraction components in the scattering pattern. The diffraction part is characterized by a rapid angular decrease of the intensity while refraction decreases moderately.

Nevertheless, the angular dependence of the spatial frequency in each of the parts of the scattering pattern is more interesting. Defining spatial frequency of a function sin[f (x)] as:

[20]

Specifically, the spatial frequency of the diffractive part:

[21] W = = 2Kr

which is a constant quantity. The refractive part, on the other hand, yields:

[22]

This relatively complex angular behavior involves the average refraction index of the examined object. This angular dependence may be exploited in the investigation of refraction index of micro particles. As refraction index grows, the dependence becomes more significant, thus enabling a quantitative extraction of optical parameters from scattering data.

Fig. 10. Measurement of polystyrene beads by microscope analysis: Experimental MIA measurements of polystyrene beads were made regarding the effect of refraction index of suspending medium on measured size. Beads were suspended in water containing different concentrations of sucrose so that relative refraction index was 0.14-0.24. Results show that this had a remarkable effect on size, measured by the automated image analysis that was used in the experiment. Vertical axis is intensity optical density horizontal stands for bead area, full circles represent beads in water and empty circles represent beads suspended in water-sucrose solution (Dn= 0.14) .